A Picture Book of Physics Theories
FEST log
Entry #008
May 19, 2024
Clarifying diagrams
Many profound discoveries in physics and mathematics were initially presented in hard to understand jargon without clear illustrations of the key points. Only years or sometimes decades later would deeper insight lead to simpler pictures, and vice versa: diagrams that were easy to interpret made it easier to get a sense of the deeper meaning of a theory.
Some profound illustrative figures in physics are Minkowski diagrams (1908) and Penrose diagrams (1963) depicting relationships in space and time. A somewhat different type is formed by Feynman diagrams (1948). In mathematics the approach of category theory is altogether based on diagrams as its building blocks.
Each of the first three examples offered profound new perspectives on the physics underlying processes related to the behavior of matter. My goal in this and following entries will be to provide diagrams that similarly shed light on the way our minds are functioning.
My initial attempts will naturally be simpler than the physics examples mentioned above, given that I am starting from scratch. My hope is that soon we can nurture a community of scientists and scholars, each with a background in science and/or contemplation, which collectively will vastly improve upon the initial ideas that I will offer here.
Matter, Experience and Appearance
As a reminder, in entry #006 I started a search for a theory, to go with the preliminary experiments 1) and 2), discussed in entry #005. I used the history of physics as the most obvious example to start with in order to get some inspiration. In entry #007 I made the prediction that whatever we find will likely be completely unexpected, and utterly different from the way we have learned to deal with our own minds in our culture.
That last suggestion is the most conservative way of extrapolating to a science of mind the way in which science of matter has shown a progression of ever more surprising discoveries. Another conservative extrapolation can be made from reading accounts of the most respected contemplatives in various traditions. The one thing they all have in common, whatever their particular tradition happened to be, is a sense of awe that is very reminiscent of the awe scientists expressed whenever they explored their own topics to greater and greater depth.
What has held back a straightforward comparison of insights in matter and mind, between scientists and contemplatives? It is that contemplatives have not yet reached agreement on any clear correspondence between their own tradition and quite different traditions. Like the prescientific state of engineering, with insights locked up within specific guilds working on specific topics, with very few exceptions no systematic attempts have been made to build real bridges, let alone to meet each other into the trenches or canyons way below such bridges.
The miracle of the science of matter was the way that a more abstract non-physical ingredient, mathematics, added to the purely physical investigations, could open doors to completely unexpected insights. Could it be that a science of mind is also still waiting for a missing ingredient? At this point we are not yet in a position to even guess what that might be. Rather than guessing, let us do some work. In other words, let's start with a working hypothesis, and take it from there.
From working hypotheses and theories to experiments
In entry #003 I introduced two working hypotheses:
WH 1: there are primitive elements underlying experience
WH 2: appearances are primitives for any form of experience
In addition, I now propose a third one:
WH 3: the shifts in perspectives between viewing objects as matter, experience, or appearance, might have analogies in the shifts of perspectives between subsequent theories in physics.
WH 3 may or may not turn out to bring us closer to a useful theory to start with. Either way, we will learn from the exercise. If it is helpful, and the analogy helps us to build such a theory, great! And if not, by seeing where the analogy fails, we are likely to at least get some hints of where else to look.
The shifts in perspective that WH 3 points are those described briefly in entry #005, corresponding to:
experiment 1): the nature of matter as experience
experiment 2): the nature of experience as appearance
There we didn't really get underway with the experiments, which would have required a more detailed description of the setups, analyses and results, as well as discussions and conclusions. We could not really do so yet, since we didn't have any theory to compare the outcomes of the experiments with. That was the reason that in the next entry, #006, we turned to physics for initial inspiration to set up a simple theory to guide the two experiments above. And now we're in a position to use WH 3 to provide a theory as a framework for performing experiments 1) and 2).
Figuring things out
Following our working hypothesis WH 3, I will present some diagrams, in a meta analogy to the three physics diagrams I mentioned at the start of this entry. Instead of mapping out physics *processes* in physical space and time, as those three diagrams did in different ways, I will present the progression of physics *perspectives* themselves diagrammatically in calendar time.
My first diagram is very simple: a straight line with two arrows. The first arrow indicates the inspiration that the Greeks received from the Babylonians, whose millennium's worth of observations of the motions of Sun, Moon and planets provided a valuable database that allowed them to construct models of the planetary system. The second arrow indicates the inspiration that Aristotle received from the Pre-Socratics, philosophers like Pythagoras, Heraclitus, Zeno and Democritus. I have added some indication of the time around which they were active.
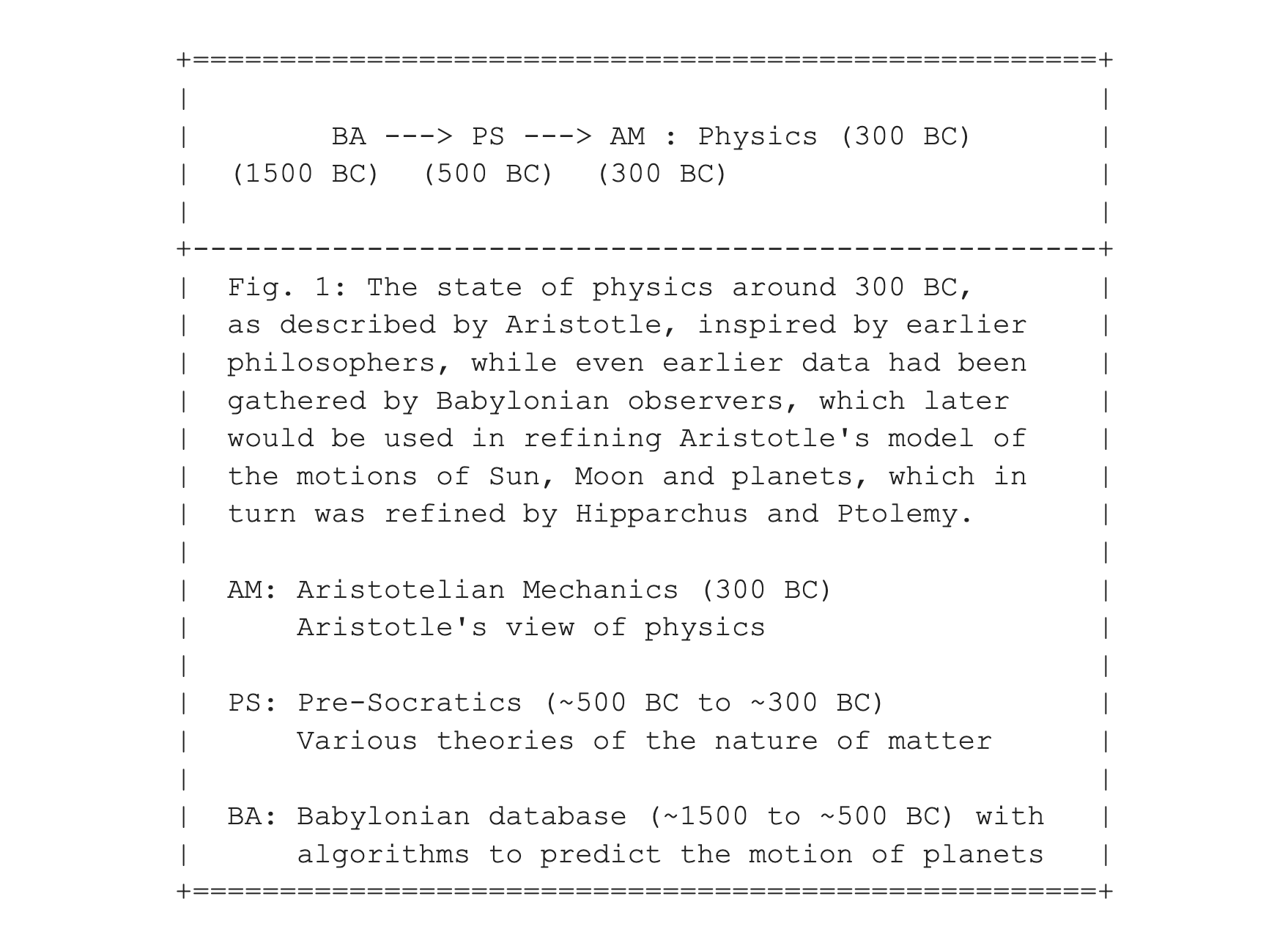
The Second diagram introduces the first modern scientific model of motions of objects that exert forces on each other, Newton's classical mechanics, CM. In addition it includes the formula for the force of gravity, as derived by Newton, also called universal gravity, UG, since it described the effects of gravity from the smallest to the largest distances then known.

Adding an extra dimension
In Fig. 2, the plus sign between CM and UG is not very informative. The reason to mention mechanics and gravity separately is that classical mechanics alone is already sufficient to describe local forces. To predict the motion of billiard balls, for example, classical mechanics as such suffices to determine their trajectories on a pool table. Only when they touch each other or touch the edge of the pool table can their motions be changed from the straight lines on which they otherwise would move.
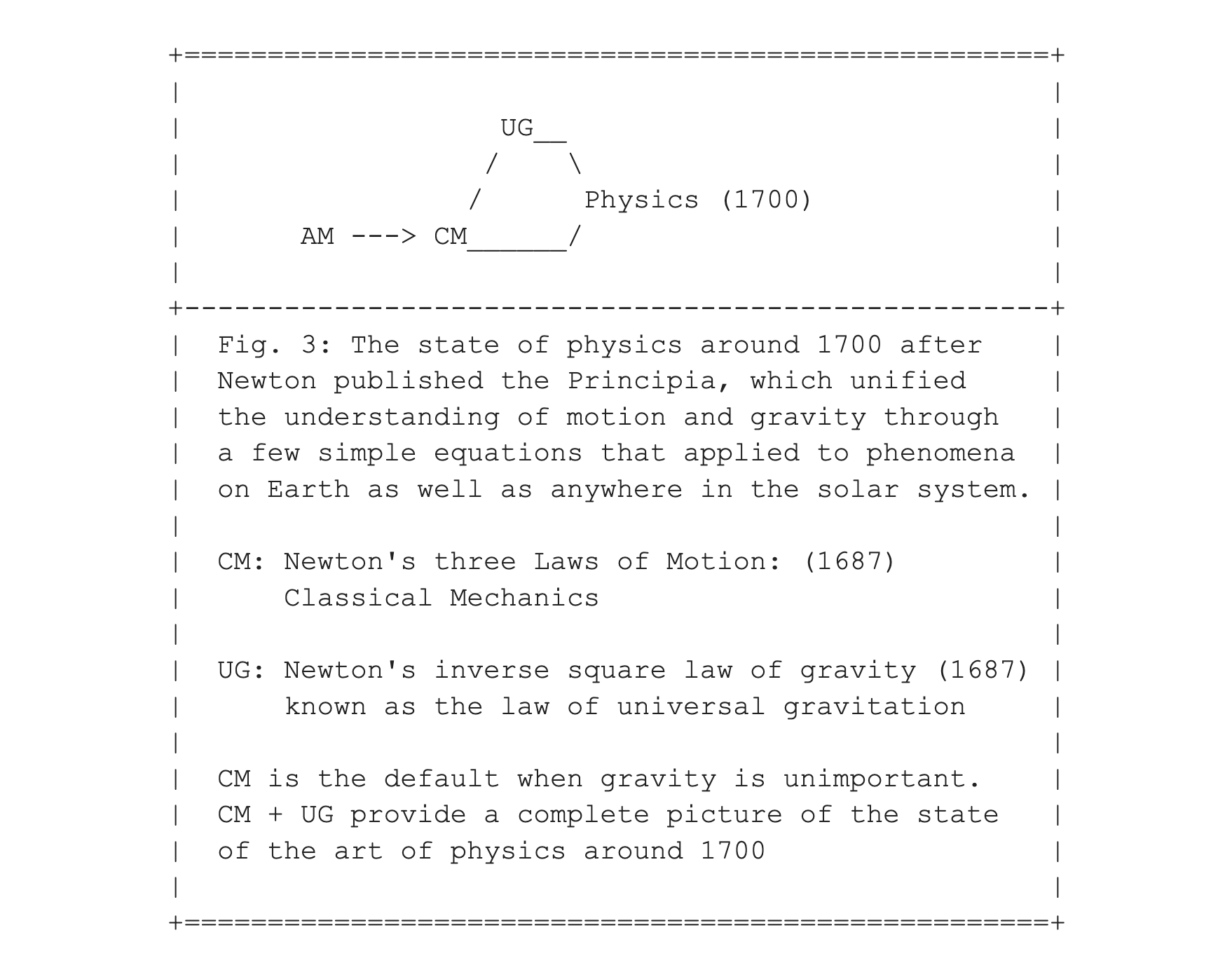
In contrast, gravity is a force that acts at a distance. Any motion of any object on or near Earth is affected by the gravitational pull that the Earth exerts on that object. To make Fig.2 into a real diagram, in Fig.3 a new dimension has been added in the vertical direction. AM -> CM now indicates how Aristotelian mechanics was replaced by Newton's classical mechanics, and how universal gravity UG added a separate embellishment, as the only force known to act in a distance.
Between Newton's classical mechanics and his universal gravity, physics was thought to be complete. This was the state of the art of physics around 1700.
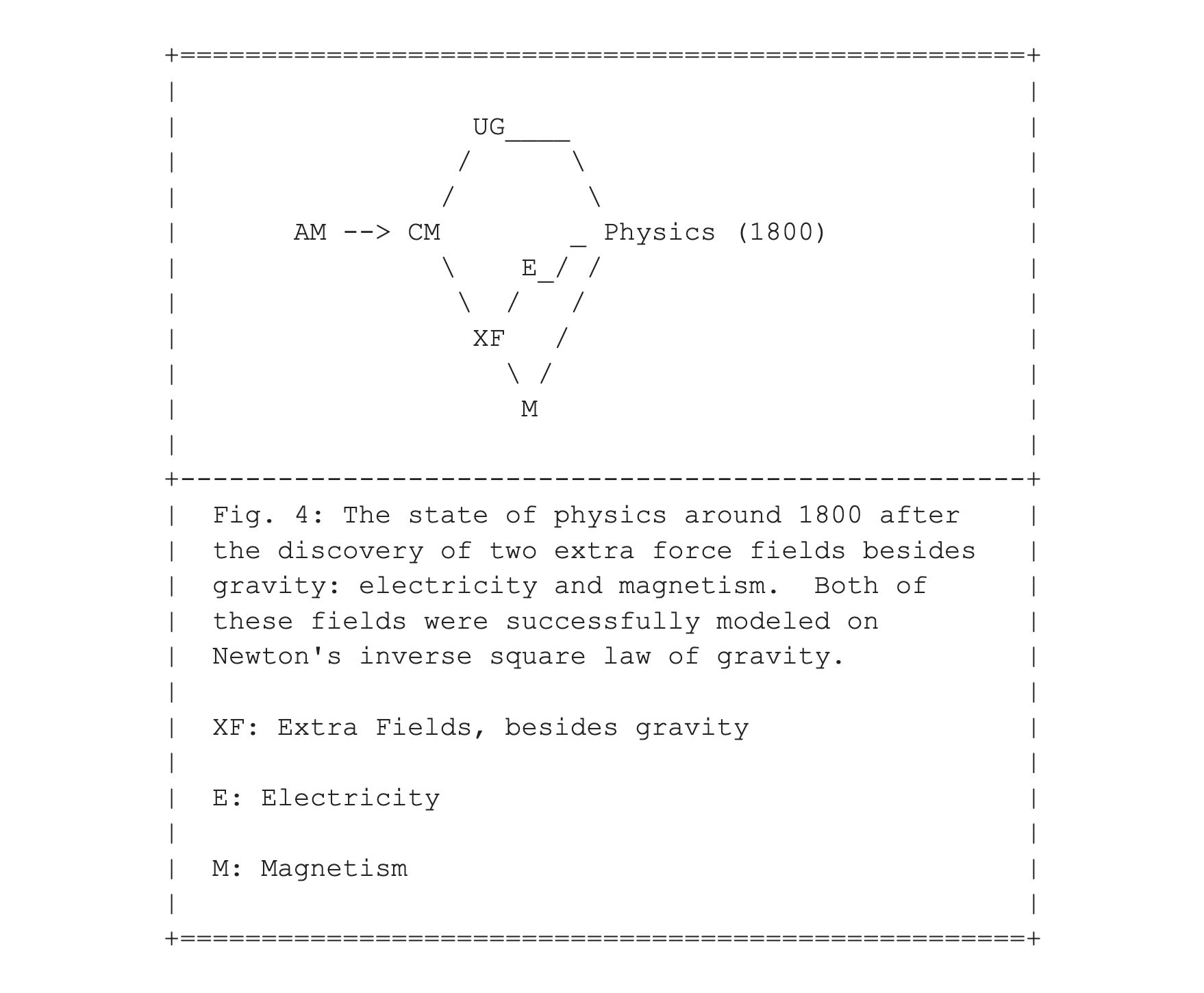
Extra forces: electricity and magnetism
Physics seemed finished and self-contained, with Newton's three laws and Newton's law of gravity. During the next century, though, two more forces were discovered to act at a distance. Not only that, they were similar in the way their forces fell off with distance, namely as the inverse square.
They were electricity and magnetism. Both were known since antiquity. Thales, one of the most prominent Pre-Socratics, described both of them, but without further detailed study. The earliest mention of the effects of electricity was already in an Egyptian text, a full two thousand years before Thales, describing how certain types of fish produced electric shocks.
The use of magnetism to build a compass was invented by the Chinese two thousand years ago, and became in use in Europe a thousand years later. William Gilbert, a contemporary of Galileo, published detailed studies of electricity and magnetism. He concluded that the Earth itself was a giant magnet, creating the Earth's magnetic field.
It was only later, in 1819, through the work of Ørsted, that relationships between electricity and magnetism became known. Fig. 4 shows the state of the art of physics, a hundred years later than Fig. 3. By then gravity had acquired two companions, also working through action at a distance, but without any clear connection between the two. Note that the simpler contact forces of CM, that are contributing to the physics of 1800, are left out for simplicity.
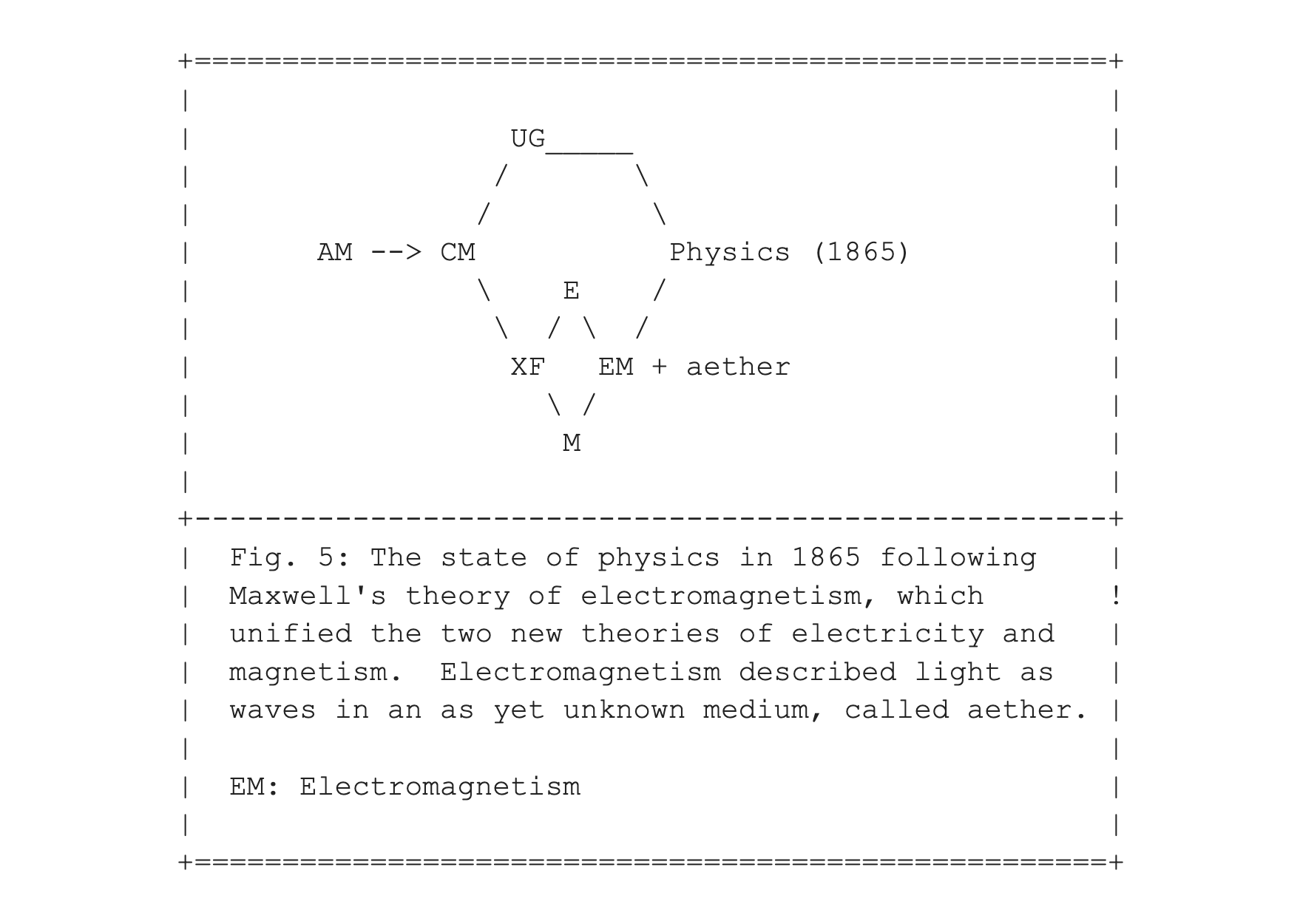
Maxwell's unification: electromagnetism
The more physicists understood the properties of electricity and magnetism and their relationships, the more it became clear that there was a strong parallel between both. There was only one glaring difference: we find electric charges in nature, but nobody has ever found magnetic "charges". Instead, a north pole and a south pole, the parallel between an electric positive and negative charge, always appear together; hence the name "poles", rather than "charges". So far, we have never found a single pole, even though we already have made up a name for one, if it were found: a magnetic monopole.
A major breakthrough was made by Maxwell's discovery of the equations of electromagnetism, a unified way to present the effects of electricity and magnetism. Apart from the absence of magnetic charges, electricity and magnetism played a very similar role in his equations. Fig. 5 shows the state of the art of physics after Maxwell's discovery.
One result of Maxwell's equations was the discovery that light is a form of electromagnetic radiation, which suggested that there would be other forms, outside the narrow wavelength band of visible light. Indeed, besides infrared and ultraviolet light, at the much longer wavelength side were radio waves, discovered by Hertz in 1888, and at the much shorter wavelength side X-rays, discovered by Röntgen in 1895.
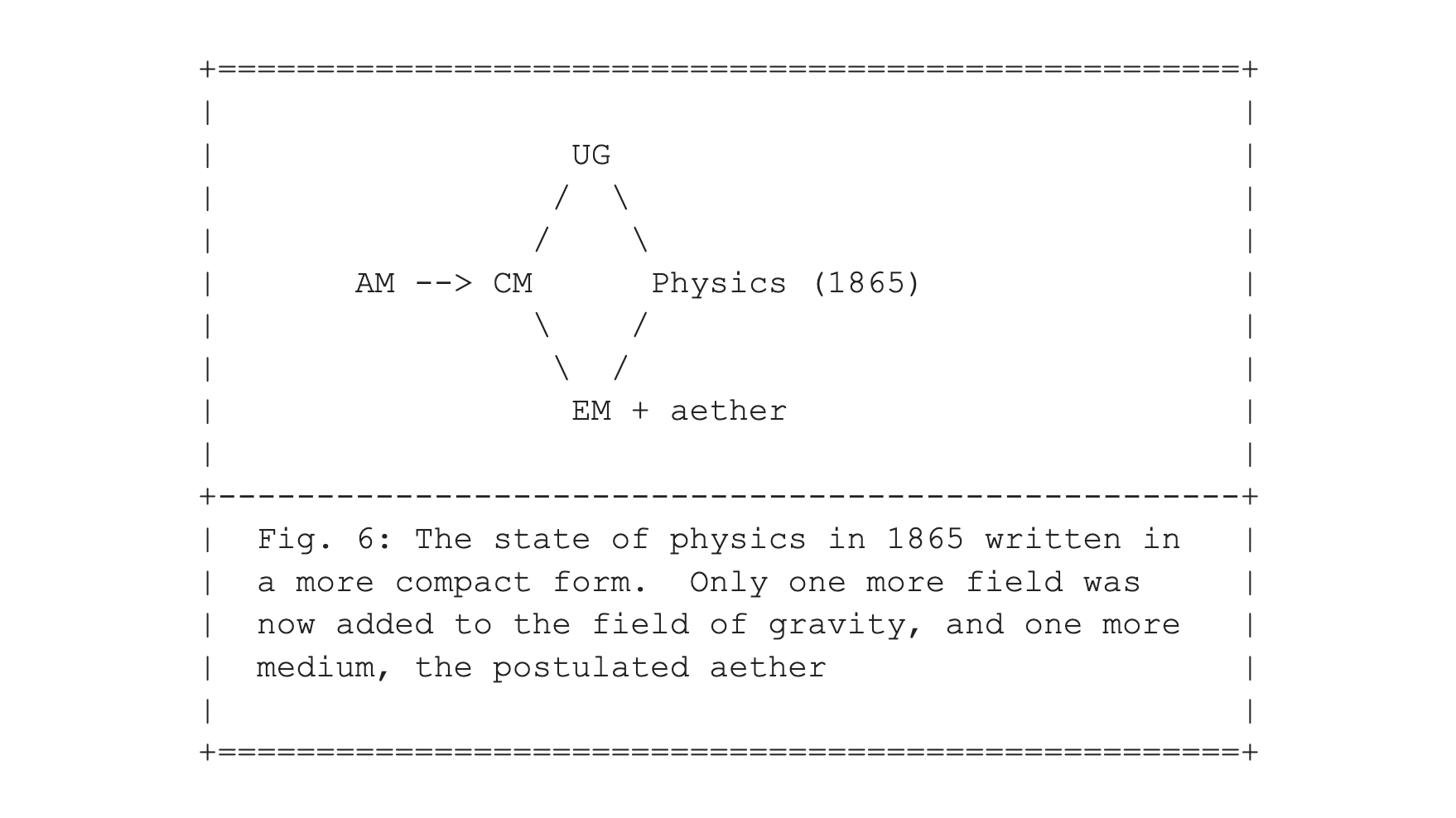
Maxwell postulated that space is filled everywhere by a very rarified medium, called aether. It was thought that any type of waves would require a medium in which to form waves in, and that it would be just a matter of time to determine the properties of that medium.
A more compact version of Fig. 5 is presented in Fig. 6, which shows a parallel with the state of physics of Fig. 3, with the first action-at-a-distant force field of gravity receiving company by a similar but more complicated field, that of electromagnetism.
A First hint from physics for creating a science of mind
Coming back to our goal of setting up, and trying out, initial theories in a science of mind, so far we can already find three hints that might turn out to be useful -- or not, we don't know yet.
First, when separate descriptions seem to be necessary for somewhat similar phenomena, there can be a payoff in terms of a simplification if we can construct a unification of two or more theories.
One example was the transition from Aristotelian to Newtonian mechanics. Where Aristotle's theory included two different realms, below and above the Moon, with completely different laws of motion, Newton's theory replaced both by a single theory.
A second example was the unification of electricity and magnetism into one single theory, showing unexpected symmetries between the two. A surprising result was that the stark difference between the presence of electric charges and the absence of magnetic monopoles turned out to be less fundamental than the way both forces played a completely symmetric role in Maxwell's equations.
A second hint
Apart from the economy and elegance of theoretical descriptions, there were more concrete payoffs as well.
In terms of the first example, the unification of gravity "up there" and "here below" immediately clarified the nature of comets, as well as the nature of tides. And ultimately it allowed us to move into the "up there" ourselves, with the first moonwalk.
As for the second example, nobody could have guessed that unification of electricity and magnetism would give an enormous bonus in understanding the nature of light, which in turn enabled the discovery of radio waves. The technological and social implications would cause a communication revolution in the early twentieth century.
A third hint
The third and last hint concerns the very nature of space and time, the stage on which physics plays out.
In the transition from Aristotle to Newton, the segregation of space into eternal motion "up there" and every motion running out of steam "down here" was abandoned. There was nothing special about the space above the Moon. Aristotle had introduced a subtle refined element, which he called aether, residing only in the heavenly realm and propelling Sun, Moon and planets in their circular orbits. With Newton space became much simpler: a sheer emptiness as an extended vacuum, no further frills needed.
In the transition from Newton to Maxwell, it seemed necessary to go back to Aristotle in spirit, and posit a new space filling material. Maxwell for convenience used the same Greek term aether. After two thousand years of Aristotle's aether and two centuries of Newton's empty vacuum, a new type of aether was introduced. We will see in the next entry that this new version only held sway for a mere forty years, till Einstein's new views of space and time showed that there was no longer a need for an aether, at least not in three dimensions. With Einstein the fundamental stage had become an altogether different one, a four dimensional spacetime, combining some space characteristics and some time characteristics into a whole new type of manifold.
More hints to come
In our picture book, we have progressed a few thousand years, up to 1865. In our next entry we will start our journey at 1900, and we will see that every 25 years from then on basic physics would go through a new and each time unexpected revolution.
However, we will also see that all that progress in theory building stopped after 1975, at least on the level of experimentally verified theory building. 2000 came and went. And soon 2025 will come, without any sign that it will not come and go as well.
It may be that the main reason is that it has become harder and harder to build ever more powerful particle accelerators. Or perhaps the next level of surprise will be out of reach by so many orders of magnitude in energy that there is no hope to make experimental progress in the foreseeable future.
Or . . . perhaps the artificial split between a science of "objective" matter and "subjective" mind no longer holds when one or both of these are reaching a limit of validity of that artificial split. We simply don't know.
For now, as a next step, let's enter the twentieth century in our next Log entry, and in the process gather a few more hints.
– Piet Hut